Posted on

Optical Surface Radius of Curvature

Optical surface radius is a primary control parameter. It must be measured to confirm the optical element meets specification. Spectrally Controlled Interferometry promises a faster and more accurate method to measure surface radius.

Multiple methods are presently used to measure radius.

  • Test plates: A test plate has a pre-measured radius to which the test surface is compared, the difference is power fringes is reported.
  • Mechanical probe: The sagitta of the lens surface is measured over a fixed diameter, from which radius is calculated. Small changes in sagitta measurement can lead to large errors in reported radii.
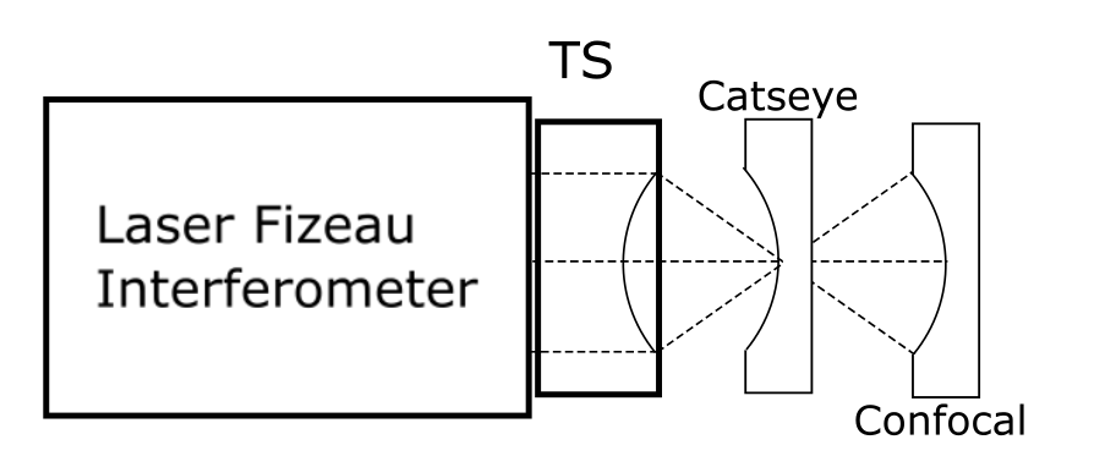
  • Interferometer “Cateye to Confocal”: An interferometer with a transmission sphere (TS) is set up at the catseye position off the surface of interest, and position noted. Then the lens is moved to the confocal position and the position noted. The difference in positions is the radius. PMI interferometers also compensate for any residual power in the measurements due to imperfect positioning. The positions are shown in figure 1.

Interferometer radius measurement is the “gold standard” method today. To achieve this measurement the following additional equipment is needed:

  • Lens mount that allows for free motion along the axis of the lens (Z axis) to micrometer position accuracy
  • Free Z motion over up to a meter, with micro-radian angular alignment to minimize cosine errors.
  • A separate Z position metrology tool. Either a glass scale or a displacement measuring interferometer (DMI) The DMI is the most accurate as it minimizes Abbe offset errors, though it adds environmental uncertainty if not calibrated.
Figure1: The distance between catseye and confocal is the surface radius. Note extra mechanical fixturing and metrology instruments are needed to measure the positions accurately

Spectrally Controlled Interferometry (SCI) Radius Measurement

SCI has a unique combination of four properties:

  • Easy alignment in laser mode
  • Electronically switch to white light mode to isolate fringes to the surface of interest
  • Measures the cavity phase electronically (no mechanical motions)
  • Measures the absolute distance of the measured cavity

SCI Absolute Position Measurement

The SCI source parameters that determine the absolute distance measurement are:

λ0 = The nominal wavelength of the SCI source
Δλ = A tunable source parameter

The absolute distance from the transmission sphere to the optical surface is:

lc = λ20 / 2Δλ   (1)

This combination means SCI can provide all the measurements required for surface radius with neither an additional DMI, nor the precision mechanics to move from Catseye to Confocal position.

SCI Radius Measurement

Since SCI can measure absolute position there is no requirement to measure at two positions. For the first time surface irregularity and radius can be measured at the same time. This also improves measurement accuracy by the elimination of several error sources as discussed below.

SCI Radius is measured by first calibrating the radius of the TS = RoCTS. Then directly measuring the cavity length lc with SCI and calculating the part RoCx from:

RoCx = RoCTS – lc   (2)

Figure 2: Measuring surface radius of SCI requires calibrating the TS radius and then directly measuring the cavity length and calculating the Test Optic radius.

Measurement Accuracy

Repeatability

The foundation of accuracy is repeatability. If a measurement process is repeatable it can then be calibrated to yield accurate results. Repeatability of measurement has been shown to be <0.5 µm for radii in the 20 mm to 175 mm range. This repeatability is better the best measurements with DMI’s.

Accuracy NIST Study

In 2001 NIST ran an experiment to determine the error sources and limits of accuracy for surface radius1. The results of that experiment and error sources are shown in figure 3, along with estimates from Typical DMI and Typical Scale (Glass) radius benches and SCI. 

A key observation is most of the error sources are due to measuring the distance between catseye and confocal. In the NIST study fully 90% of the errors are in this part of the measurement. The same is true for the DMI and Glass Scale. The only difference is the magnitude of the errors that are 16X and nearly 500X greater, respectively, than the NIST study. 

These values are a precaution to users of a DMI or glass scale radius bench to not underestimate the errors in the measurements.

Figure 3: Surface radius error budget for several methods of measurement.

SCI Eliminates the Z Axis Motion Error Sources

No Z motion eliminates the first six error sources (green). Leaving the balance of the error sources that are equal to the Typical DMI set up. SCI can potentially improve the uncertainty to better than the NIST study.

An additional error source is the calibration of the TS radius that also must be added. Since it can be bootstrapp calibrated to the same level a simple doubling of the errors to ~50 nm is expected. 

As of October 2020 SCI can measure surface radius up to 250 nm cavity lengths, and potentially out to 500 mm cavity lengths. 

Summary

SCI promises to replace traditional catseye-confocal lens surface radius measurement by saving time and greatly improving accuracy. It is the go to method for high-performance lens radius metrology.

1 T. Schmitz, A. Davies, C. Evans; Uncertainties in interferometric measurements of radius of curvature, Appeared in Optical Manufacturing and Testing IV, H. Philip Stahl, Editor, Proceedings of SPIE Vol. 4451, 432-447, 2001

Posted on

Measuring Material Homogeneity with SCI

Material Homogeneity

Optical index homogeneity is an important material parameter influencing lens performance. Laser interferometers have painstakingly measured this parameter which requires four separate measurements.  Laser interferometers have either required the use of contact plates with oft times poisonous index matching fluids. Or polishing a wedge into the test part to isolate the front from the back surface. In both cases the test part must be repeatedly tilted to isolate from test parts front and back surfaces as shown in figure 1. Tilting lowers the measurement accuracy as the test beams do not return over themselves (common path operation is lost), makes the test more complicated, and slows the measurement down exposing the test part to thermal variations increasing measurement uncertainty.

figure 1: Four measurements are required to measure homogeneity. With a laser source the test part must be tilted twice adding measurement errors and complicating the measurement.

Laser Wavelength Scanning Fourier Interferometer

Recently laser wavelength scanning Fourier (LWSF)  interferometry has been used for homogeneity measurements. LWSF enables the test part to be plane parallel polished and set up where no tilting during the measurement sequence is required. Once set up two measurement sets are required, one with the test part and one without. LWSF takes up to a minute to measure the cavity, therefore it exposes the measurement to environmental changes, limiting repeatability and accuracy. Plus vibration tolerant phase algorithms are not applicable.

LWSF Surface Confusion

Plus there can be confusion as to what surfaces are measured since the Fourier algorithm “finds” the six sets of fringes present in the cavity of which only four are of interest. 

SCI Simplifies Homogeneity Measurements for Improved Accuracy

SCI, similar to LWSF, uses a plane parallel polished test part to measure homogeneity. Therefore the test part is easy to manufacture. In the laser alignment mode SCI enables easy alignment of the four surfaces. Starting with the empty cavity nulled alignment, then adding the test part and minimizing fringes to complete alignment. 

With SCI only the cavity to be measured is seen, there is no confusion. After setting up, switch to the short coherence, white light, mode:

  1. Select the transmission flat (TF) to test part S1. Measure with vibration tolerant (VT) PSI in < 1 second.
  2. Then electronically select TF to S2 and measure with VTPSI. 
  3. Now select the TF to RF cavity – through the test part – measure. 
  4. Finally remove the test part and measure the TF to RF cavity. 

REVEAL homogeneity application leads the user through these sets, see figure 2. 

figure 2: REVEAL leads the user step by step through the process. Enable the next measurement to be made and proceed.

Once the four measurements are complete the homogeneity result computed automatically, see figure 3.

figure 3: The final result of four measurements with the homogeneity mapped on the right and PV result of 19.107 ppm shown in the middle bottom.

See the link below for a ÄPRE technical paper regarding the computing the uncertainty of measurement of homogeneity measurements.

Summary

Homogeneity is an important performance parameter for optical materials. Spectrally Controlled Interferometry (SCI) simplifies the measurement, improves accuracy and ÄPRE REVEAL software leads the user step by step through the process.

Homogeneity Measurement Uncertainty Technical Paper >

More Applications >

Learn More about SCI>

Applied Optics Paper >

Let’s Talk>